Auteur/autrice : davidlannes

M. Rigal, P. Bonneton, D. Lannes. Download

We present a novel approach to handle open boundary conditions for a Boussinesq-
type wave model coupled with the nonlinear shallow water equations. Traditional
methods for managing open boundaries — such as sponge layers and source
functions — are computationally intensive and require ad hoc calibration. To
address this, we reformulate the Boussinesq equations as a system of conservation
laws with nonlocal flux and a rapidly decaying source term. This reformulation is
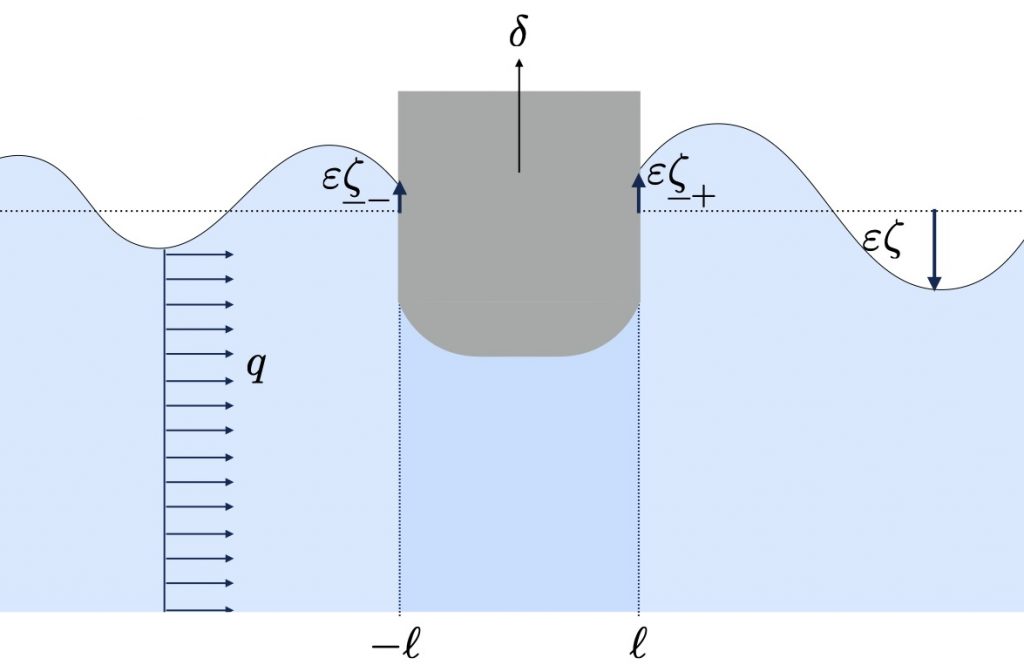
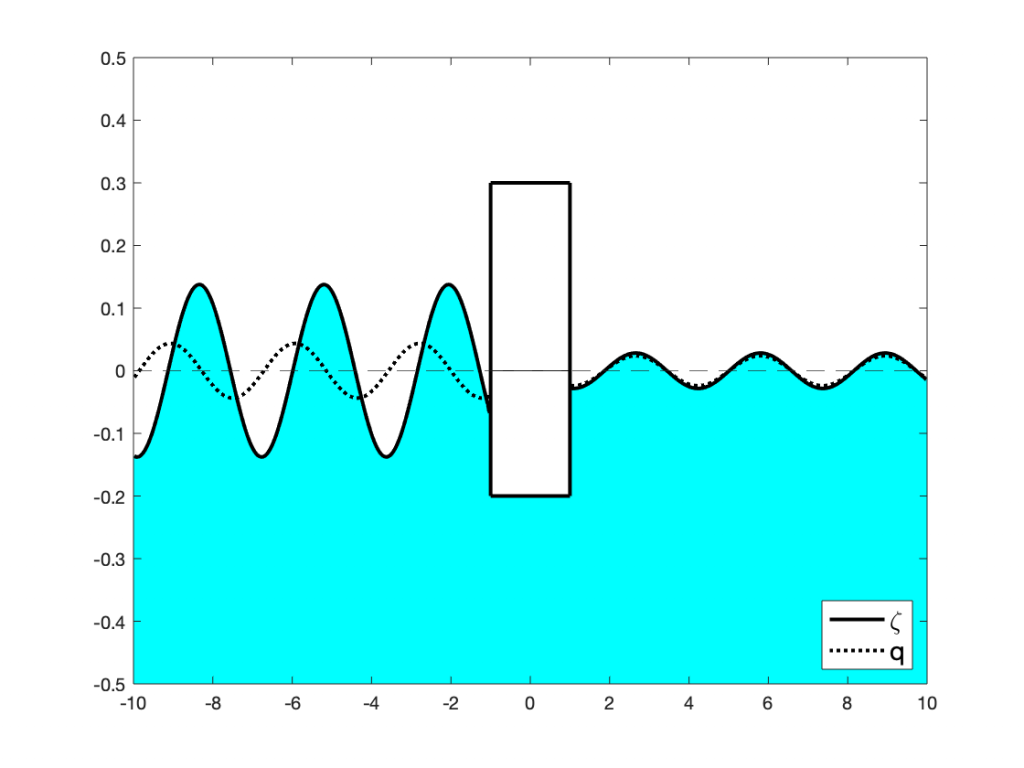
adapted to generate waves at the boundary of the numerical domain, from surface
elevation data in situations where both incoming and outgoing waves are present.
The proposed numerical scheme employs a MacCormack prediction-correction
strategy combined with finite volume and finite difference methods, preserving
key physical properties and ensuring stability. Comparison with laboratory
experiments demonstrates that our approach avoids boundary reflection issues.
In particular, it is able to accurately reproduce infragravity waves associated
with a random wave field propagating over a sloping beach. This work opens
important perspectives for improving phase-resolving coastal wave models, with
the aim of forecasting complex random wave conditions in natural environments

T. Iguchi, D. Lannes, , https://arxiv.org/pdf/2501.

We consider the initial value problem for a nonlinear shallow water model in horizontal dimension d = 2 and in the presence of a fixed partially immersed solid body on the water surface. We assume that the bottom of the solid body is the graph of a smooth function and part of it is in contact with the water. As a result, we have a contact line where the solid body, the water, and the air meet. In our setting of the problem, the projection of the contact line on the horizontal plane moves freely due to the motion of the water surface even if the solid body is fixed. This wave-structure interaction problem reduces to an initial boundary value problem for the nonlinear shallow water equations in an exterior domain with a free boundary, which is the projection of the contact line. The objective of this paper is to derive a priori energy estimates locally in time for solutions at the quasilinear regularity threshold under assumptions that the initial flow is irrotational and subcritical and that the initial water surface is transversal to the bottom of the solid body at the contact line. The key ingredients of the proof are the weak dissipativity of the system, the introduction of second order Alinhac good unknowns associated with a regularizing diffeomorphism, and a new type of hidden boundary regularity for the nonlinear shallow water equations. This last point is crucial to control the regularity of the contact line; it is obtained by combining the use of the characteristic fields related to the eigenvalues of the boundary matrix together with Rellich type identities.

T. Iguchi, D. Lannes, J. European Math. Soc, (2025). Download

This article is devoted to the proof of the well-posedness of a model describing waves propagating in shallow water in horizontal dimension d=2 and in the presence of a fixed partially immersed object. We first show that this wave-interaction problem reduces to an initial boundary value problem for the nonlinear shallow water equations in an exterior domain, with boundary conditions that are fully nonlinear and nonlocal in space and time. This hyperbolic initial boundary value problem is characteristic, does not satisfy the constant rank assumption on the boundary matrix, and the boundary conditions do not satisfy any standard form of dissipativity. Our main result is the well-posedness of this system for irrotational data and at the quasilinear regularity threshold. In order to prove this, we introduce a new notion of weak dissipativity, that holds only after integration in time and space. This weak dissipativity allows higher order energy estimates without derivative loss; the analysis is carried out for a class of linear non-characteristic hyperbolic systems, as well as for a class of characteristic systems that satisfy an algebraic structural property that allows us to define a generalized vorticity. We then show, using a change of unknowns, that it is possible to transform the linearized wave-interaction problem into a non-characteristic system, which satisfies this structural property and for which the boundary conditions are weakly dissipative. We can therefore use our general analysis to derive linear, and then nonlinear, a priori energy estimates. Existence for the linearized problem is obtained by a regularization procedure that makes the problem non-characteristic and strictly dissipative, and by the approximation of the data by more regular data satisfying higher order compatibility conditions for the regularized problem. Due to the fully nonlinear nature of the boundary conditions, it is also necessary to implement a quasilinearization procedure. Finally, we have to lower the standard requirements on the regularity of the coefficients of the operator in the linear estimates to be able to reach the quasilinear regularity threshold in the nonlinear well-posedness result.

A. Filippini, L. Arpaia, V. Perrier, R. Pedreros, P. Bonneton, D. Lannes, F. Marche, S. De Brye, S. Delmas, S. Lecacheux, F. Boulahya, M. Ricchiuto, Ocean Modelling 192 (2024), 102447. Download.

Hydrodynamic modeling for coastal flooding risk assessment is a highly relevant topic. Many operational tools available for this purpose use numerical techniques and implementation paradigms that reach their limits when confronted with modern requirements in terms of resolution and performances. In this work, we present a novel operational tool for coastal hazards predictions, currently employed by the BRGM agency (the French Geological Survey) to carry out its flooding hazard exposure studies and coastal risk prevention plans on International and French territories. The model, called UHAINA (wave in the Basque language), is based on an arbitrary high-order discontinuous Galerkin discretization of the nonlinear shallow water equations with SSP Runge–Kutta time stepping on unstructured triangular grids. It is built upon the finite element library AeroSol, which provides a modern C++ software architecture and high scalability, making it suitable for HPC applications. The paper provides a detailed development of the mathematical and numerical framework of the model, focusing on two key-ingredients : (i) a pragmatic
treatment of the solution in partially dry cells which guarantees efficiently well-balancedness, positivity and mass conservation at any polynomial order; (ii) an artificial viscosity method based on the physical dissipation of the system of equations providing nonlinear stability for non-smooth solutions. A set of numerical validations on academic benchmarks is performed to highlight the efficiency of these approaches. Finally, UHAINA is applied on a real operational case of study, demonstrating very satisfactory results.

D Lannes, M Rigal

Studies in Applied Mathematics 153 (4), e12751. Download

This paper is devoted to the theoretical and numeri-
cal investigation of the initial boundary value problem
for a system of equations used for the description of
waves in coastal areas, namely, the Boussinesq–Abbott
system in the presence of topography. We propose a pro-
cedure that allows one to handle very general linear
or nonlinear boundary conditions. It consists in reduc-
ing the problem to a system of conservation laws with
nonlocal fluxes and coupled to an ordinary differen-
tial equation. This reformulation is used to propose two
hybrid finite volumes/finite differences schemes of first
and second order, respectively. The possibility to use
many kinds of boundary conditions is used to investigate
numerically the asymptotic stability of the boundary
conditions, which is an issue of practical relevance in
coastal oceanography since asymptotically stable bound-
ary conditions would allow one to reconstruct a wave
field from the knowledge of the boundary data only, even
if the initial data are not known.

D Lannes, M Ming, https://arxiv.org/pdf/2407.18082

The goal of this paper is to prove the well-posedness of F. John’s floating body problem in the case of a fixed object and for unsteady waves, in horizontal dimension $d=1$ and with a possibly emerging bottom. This problem describes the interactions of waves with a partially immersed object using the linearized Bernoulli equations. The fluid domain $\Omega$ therefore has corners where the object meets the free surface, which consists of various connected components. The energy space associated with this problem involves the space of traces on these different connected components of all functions in the Beppo-Levi space $\dot{H}^1(\Omega)$; we characterize this space, exhibiting non local effects linking the different connected components. We prove the well-posedness of the Laplace equation in corner domains, with mixed boundary conditions and Dirichlet data in this trace space, and study several properties of the associated Dirichlet-Neumann operator (self-adjointness, ellipticity properties, etc.). This trace space being only semi-normed, we cannot use standard semi-group theory to solve F. John’s problem: one has to choose a realization of the homogeneous space (i.e. choose an adequate representative in the equivalence class) we are working with. When the fluid domain is bounded, this realization is obtained by imposing a zero-mass condition; for unbounded fluid domains, we have to choose a space-time realization which can be interpreted as a particular choice of the Bernoulli constant. Well-posedness in the energy space is then proved. Conditions for higher order regularity in times are then derived, which yield some limited space regularity that can be improved through smallness assumption on the contact angles. We finally show that higher order regularity away from the contact points can be achieved through weighted estimates.

D. Lannes, Lecture notes volume of the Winter School on Fluid Dynamics, Dispersive Equations and Quantum Fluids (Bressanone, december 2018), to appear. Download

The goal of these notes is to point out similarities and differences between two kinds of initial boundary value problems in dimension one. The first one concerns hyperbolic systems (such as the nonlinear shallow water equations) while the second one concerns dispersive perturbations of such systems (such as Boussinesq systems). In the absence of a boundary, that is, for the initial value problem, the link between both classes is quite obvious but in the presence of a boundary, the situation is more complex and dispersive boundary layers must be understood if one wants to understand the links between both classes of problems. After reviewing several types of initial boundary value problems (some of which being free boundary problems) arising in the study of waves in shallow water, we sketch the general theory for hyperbolic initial boundary value problems developed in [18] and that encompasses all of the above examples that involve hyperbolic systems. Such a general theory does not exist for dispersive perturbations of hyperbolic systems, but we treat two important examples involving Boussinesq systems. In the first one, we show that the nature of the initial boundary value problem shares little in common with the hyperbolic configuration. For instance, the problem has the structure of an ODE and no higher order compatibility conditions on the data are required to have solutions of high regularity. These differences naturally raise the questions of the control of the time of existence and of the dispersionless limit; they are addressed in a second example motivated by a wave-structure interaction problem. We explain the approach developed in [13] to treat this problem, pointing out the role played by dispersive boundary layers.