G. Beck, D. Lannes, L. Weynans, arxiv.org/abs/2307.01749, Download
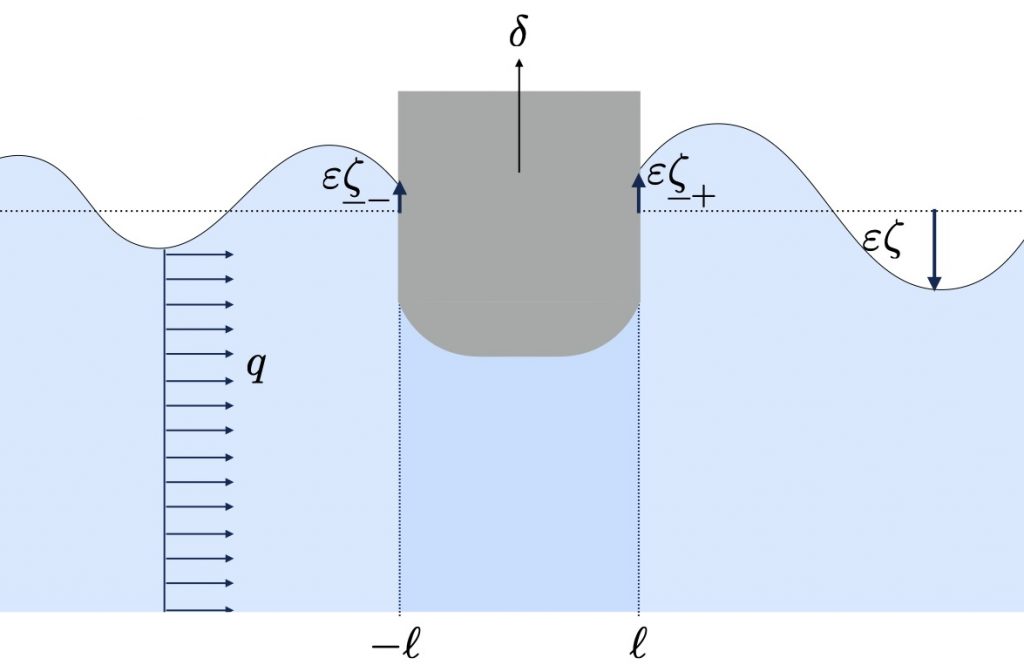
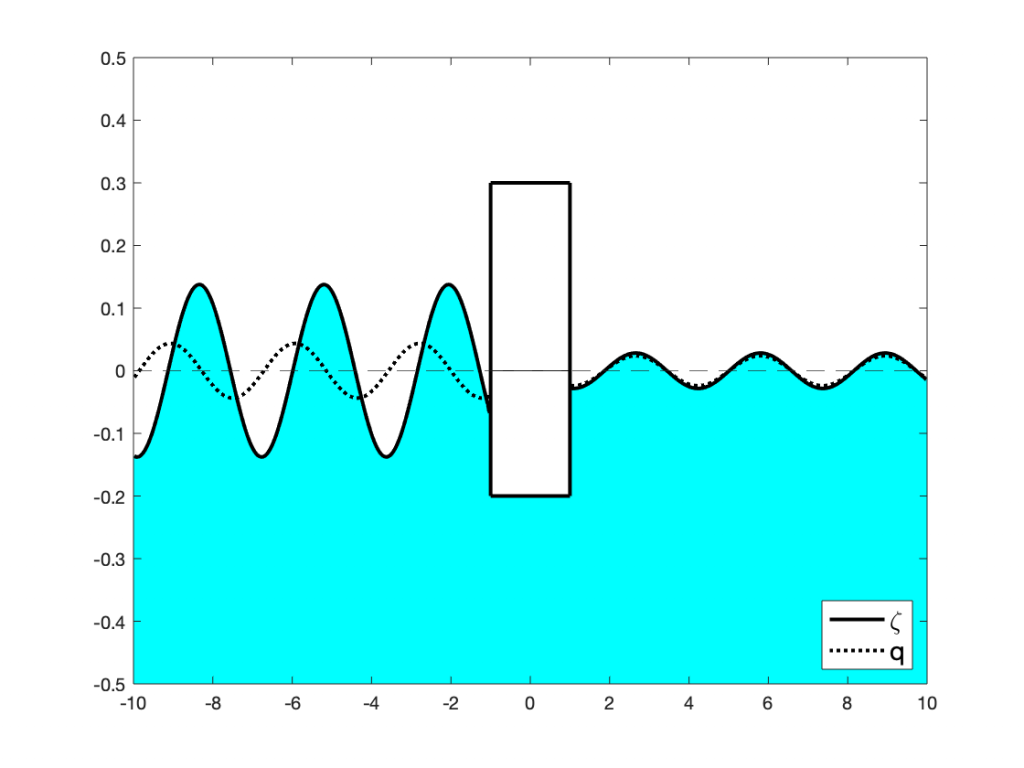
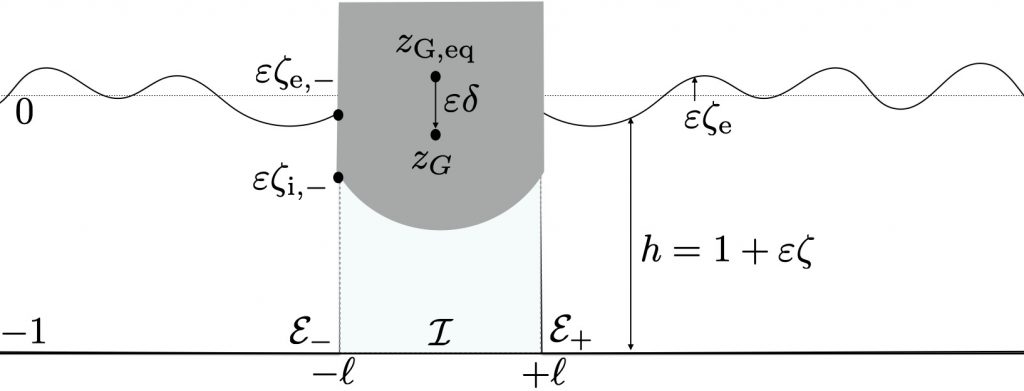
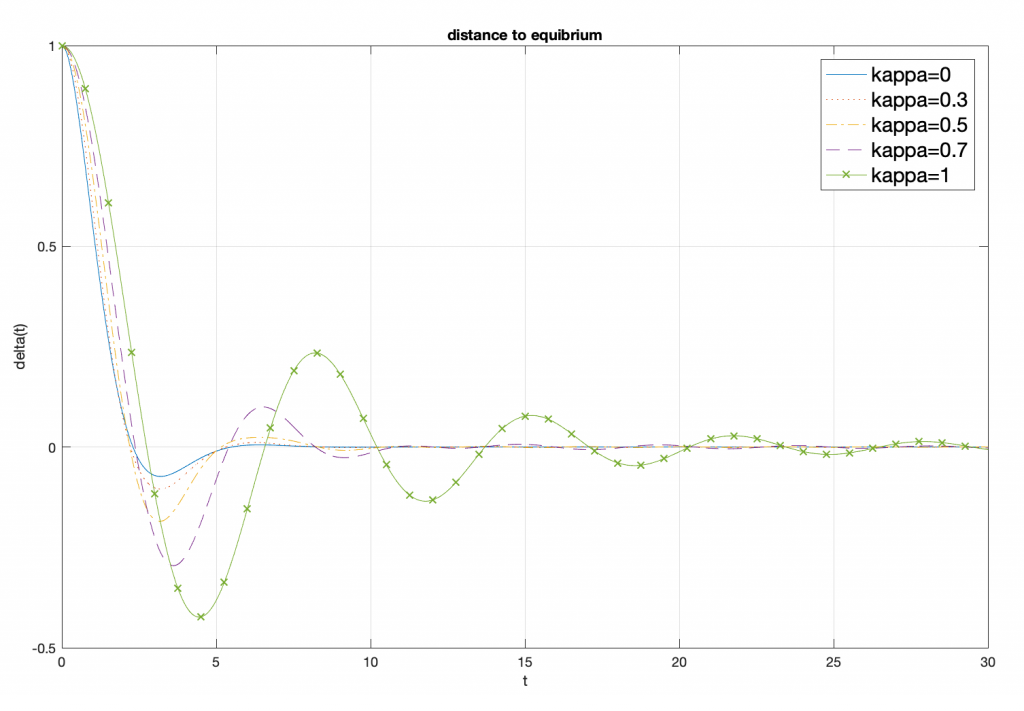
The goal of this work is to study waves interacting with partially immersed objects allowed to move freely in the vertical direction, and in a regime in which the propagation of the waves is described by the one dimensional Boussinesq-Abbott system. The problem can be reduced to a transmission problem for this Boussinesq system, in which the transmission conditions between the components of the domain at the left and at the right of the object are determined through the resolution of coupled forced ODEs in time satisfied by the vertical displacement of the object and the average discharge in the portion of the fluid located under the object. We propose a new extended formulation in which these ODEs are complemented by two other forced ODEs satisfied by the trace of the surface elevation at the contact points. The interest of this new extended formulation is that the forcing terms are easy to compute numerically and that the surface elevation at the contact points is furnished for free. Based on this formulation, we propose a second order scheme that involves a generalization of the MacCormack scheme with nonlocal flux and a source term, which is coupled to a second order Heun scheme for the ODEs. In order to validate this scheme, several explicit solutions for this wave-structure interaction problem are derived and can serve as benchmark for future codes. As a byproduct, our method provides a second order scheme for the generation of waves at the entrance of the numerical domain for the Boussinesq-Abbott system.