Catégorie : Recent

Waves interacting with a partially immersed obstacle in the Boussinesq regime

13 février 2019 / davidlannes

D. Bresch, D. Lannes, G. Métivier, to appear in Analysis & PDE, Analysis & PDE, 14 (2021), 1085-1124 Download

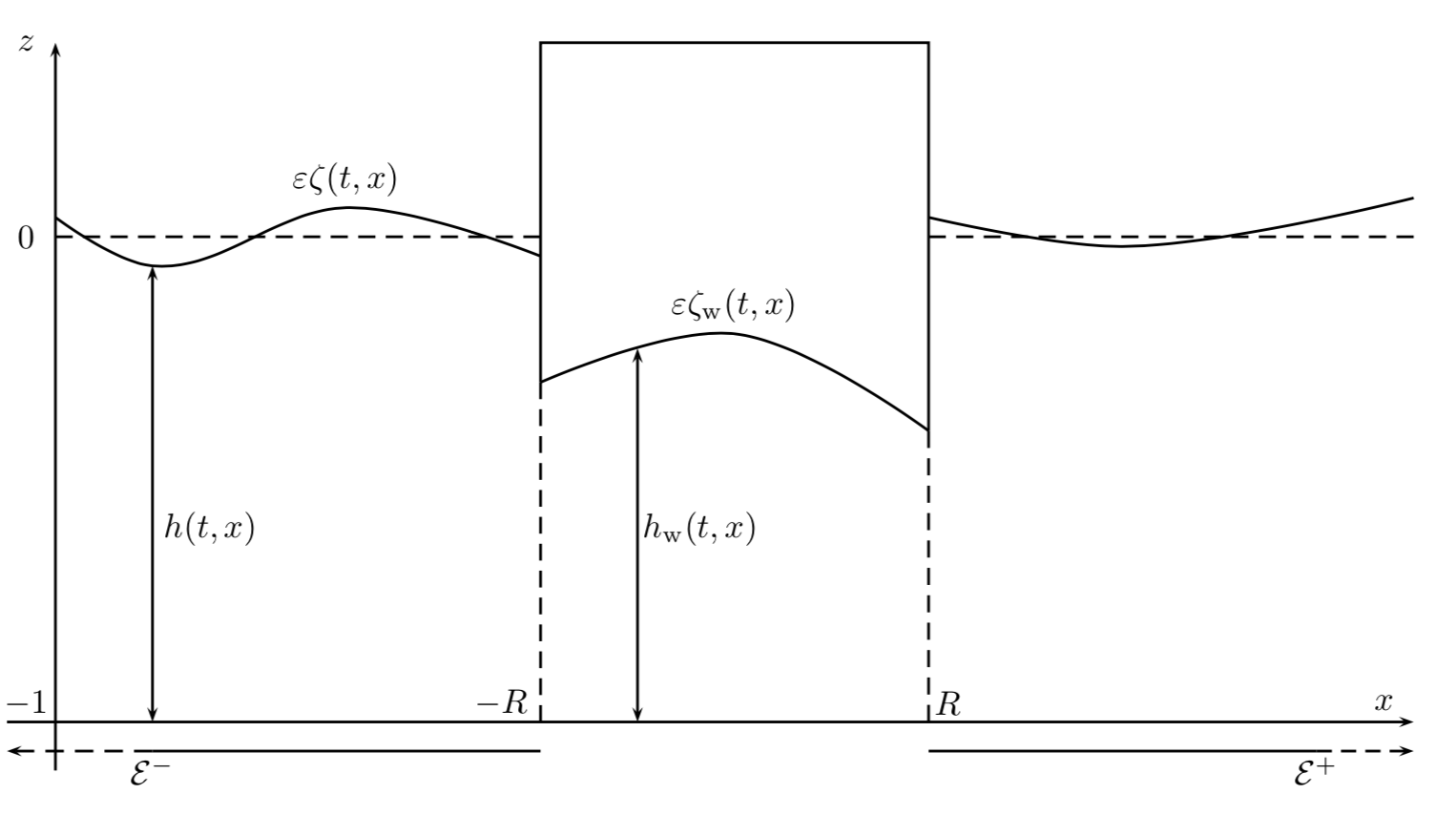
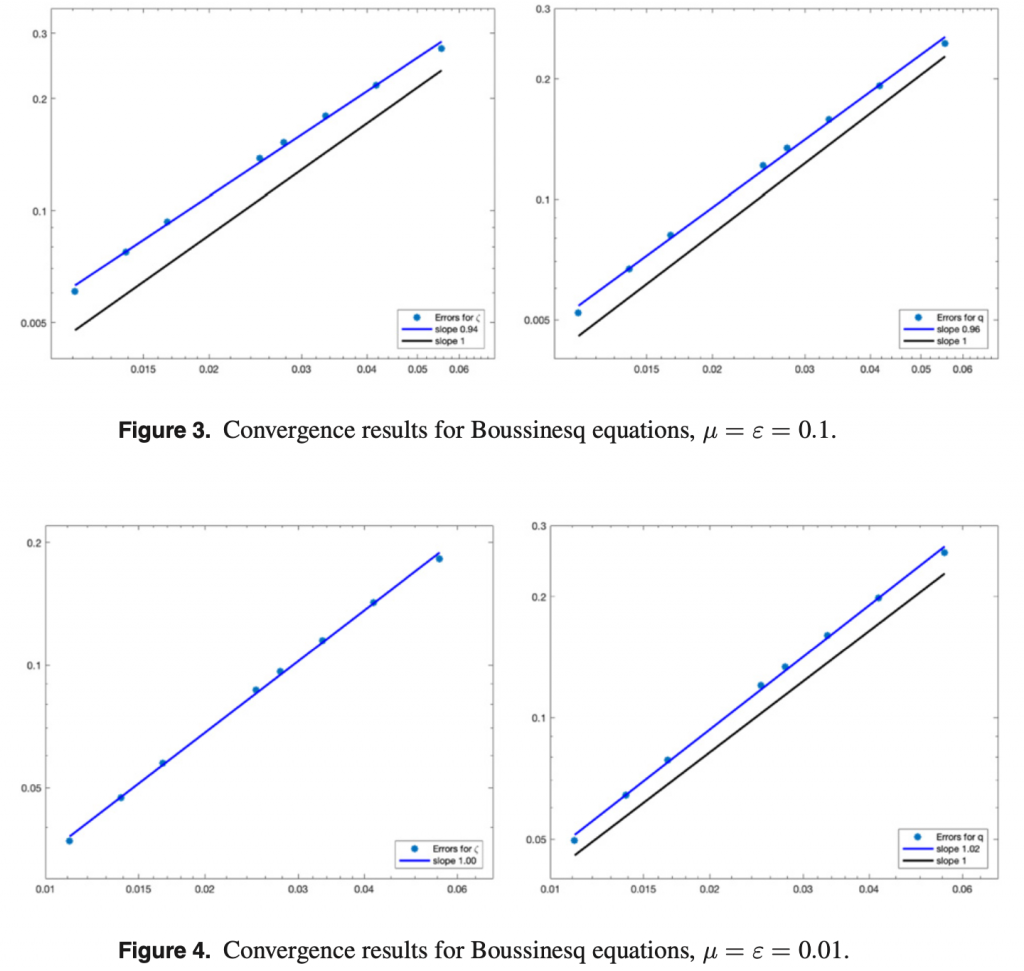
This paper is devoted to the derivation and mathematical analysis of a wave-structure interaction problem which can be reduced to a transmission problem for a Boussinesq system. Initial boundary value problems and transmission problems in dimension d= 1 for 2 x 2 hyperbolic systems are well understood. However, for many applications, and especially for the description of surface water waves, dispersive perturbations of hyperbolic systems must be considered. We consider here a conguration where the motion of the waves is governed by a Boussinesq system (a dispersive perturbation of the hyperbolic nonlinear shallow water equations), and in the presence of a fixed partially immersed obstacle. We shall insist on the differences and similarities with respect to the standard hyperbolic case, and focus our attention on a new phenomenon, namely, the apparition of a dispersive boundary layer. In order to obtain existence and uniform bounds on the solutions over the relevant time scale, a control of this dispersive boundary layer and of the oscillations in time it generates is necessary. This analysis leads to a new notion of compatibility condition that is shown to coincide with the standard hyperbolic compatibility conditions when the dispersive parameter is set to zero. To the authors’ knowledge, this is the rst time that these phenomena (likely to play a central role in the analysis of initial boundary value problems for dispersive perturbations of hyperbolic systems) are exhibited.

Generating boundary conditions for a Boussinesq system

3 février 2019 / davidlannes

D. Lannes, L. Weynans, Nonlinearity 33 (2020), 6868 Downl oad

We present a new method for the numerical implementation of generating boundary conditions for a one dimensional Boussinesq system. This method is based on a reformulation of the equations and a resolution of the dispersive boundary layer that is created at the boundary when the boundary conditions are non homogeneous. This method is implemented for a simple first order finite volume scheme and validated by several numerical simulations. Contrary to the other techniques that can be found in the literature, our approach does not cause any increase in computational time with respect to periodic boundary conditions

Hyperbolic free boundary problems and applications to wave-structure interactions

31 janvier 2019 / davidlannes

T. Iguchi, D. Lannes, to appear in Indiana University Journal of Mathematics, Download

Motivated by a new kind of initial boundary value problem (IBVP) with a free boundary arising in wave-structure interaction, we propose here a general approach to one dimensional IBVP as well as transmission problems. For general strictly hyperbolic N xN
quasilinear hyperbolic systems, we derive new sharp linear estimates with refined dependence on the source term and control on the traces of the solution at the boundary. These new estimates are used to obtain sharp results for quasilinear IBVP and transmission problems, and we also use them to propose a general approach to 2 x2 quasilinear IBVP and transmission problems with a moving or possibly free boundary. In the latter case, two kinds of evolution equations for the boundary are considered. The first one is of « kinematic type » in the sense that the velocity of the interface has the same regularity as the trace of the solution. Several applications that fall into this category are considered: the interaction of waves with a lateral piston, and a new version of the well-known stability of shocks (classical and undercompressive) that improves the results of the general theory by taking advantage of the specificities of the one-dimensional case. We also consider « fully nonlinear » evolution equations characterized by the fact that the velocity of the interface is one derivative more singular than the trace of the solution. This configuration is the most challenging; it is motivated by a free boundary problem arising in wave-structure interaction, namely, the evolution of the contact line between a floating object and the water. This problem is solved as an application of the general theory developed here.

An example of free boundary with kinematic boundary condition: the piston problem

An example of free boundary with fully nonlinear boundary condition : the floating structure

The shoreline problem for the one-dimensional shallow water and Green- Naghdi equations

16 janvier 2019 / davidlannes

D. Lannes, G. Métivier, J. Ec. Polytech. Math. 5 (2018), 455–518. Download

The Green-Naghdi equations are a nonlinear dispersive perturbation of the nonlinear shallow water equations, more precise by one order of approximation. These equations are commonly used for the simulation of coastal flows, and in particular in regions where the water depth vanishes (the shoreline). The local well-posedness of the Green-Naghdi equations (and their justification as an asymptotic model for the water waves equations) has been extensively studied, but always under the assumption that the water depth is bounded from below by a positive constant. The aim of this article is to remove this assumption. The problem then becomes a free-boundary problem since the position of the shoreline is unknown and driven by the solution itself. For the (hyperbolic) nonlinear shallow water equation, this problem is very related to the vacuum problem for a compressible gas. The Green-Naghdi equation include additional nonlinear, dispersive and topography terms with a complex degenerate structure at the boundary. In particular, the degeneracy of the topography terms makes the problem loose its quasilinear structure and become fully nonlinear. Dispersive smoothing also degenerates and its behavior at the boundary can be described by an ODE with regular singularity. These issues require the development of new tools, some of which of independent interest such as the study of the mixed initial boundary value problem for dispersive perturbations of characteristic hyperbolic systems, elliptic regularization with respect to conormal derivatives, or general Hardy-type inequalities.

A nonlinear weakly dispersive method for recovering the elevation of irrotational surface waves from pressure measurements

1 août 2018 / davidlannes

P. Bonneton, D. Lannes, K. Martins, H. Michallet, Coastal Engineering 138 (2018), 1–8. Download

We present the derivation of a nonlinear weakly dispersive formula to reconstruct, from pressure measurements, the surface elevation of nonlinear waves propagating in shallow water. The formula is simple and easy to use as it is local in time and only involves first and second order time derivatives of the measured pressure. This novel approach is evaluated on laboratory and field data of shoaling waves near the breaking point. Unlike linear methods, the nonlinear formula is able to reproduce at the individual wave scale the peaked and skewed shape of nonlinear waves close to the breaking point. Improvements in the frequency domain are also observed as the new method is able to accurately predict surface wave elevation spectra over four harmonics. The nonlinear weakly dispersive formula derived in this paper represents an economic and easy to use alternative to direct wave elevation measurement methods (e.g. acoustic surface tracking and LiDAR scanning).